DC Analytic Dipole

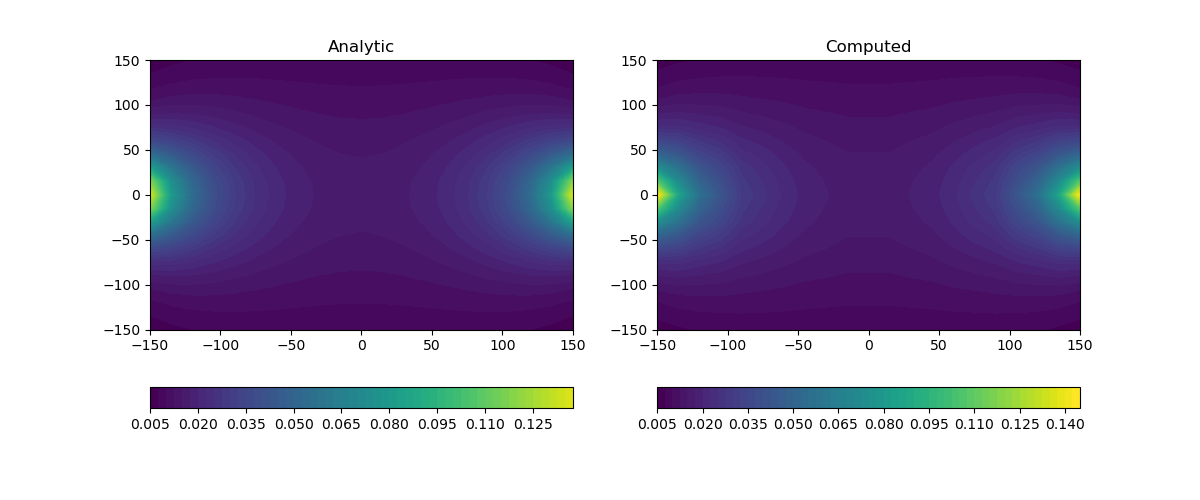
Comparison of the analytic and numerical solution for a direct current resistivity dipole in 3D.

/home/vsts/work/1/s/simpeg/base/pde_simulation.py:490: DefaultSolverWarning:

Using the default solver: Pardiso.

If you would like to suppress this notification, add
warnings.filterwarnings('ignore', simpeg.utils.solver_utils.DefaultSolverWarning)
 to your script.

/usr/share/miniconda/envs/simpeg-test/lib/python3.10/site-packages/pymatsolver/solvers.py:415: FutureWarning:

In Future pymatsolver v0.4.0, passing a vector of shape (n, 1) to the solve method will return an array with shape (n, 1), instead of always returning a flattened array. This is to be consistent with numpy.linalg.solve broadcasting.

0.06464333484677044

import discretize
from simpeg import utils
import numpy as np
import matplotlib.pyplot as plt
from simpeg.electromagnetics.static import resistivity as DC


cs = 25.0
hx = [(cs, 7, -1.3), (cs, 21), (cs, 7, 1.3)]
hy = [(cs, 7, -1.3), (cs, 21), (cs, 7, 1.3)]
hz = [(cs, 7, -1.3), (cs, 20)]
mesh = discretize.TensorMesh([hx, hy, hz], "CCN")
sighalf = 1e-2
sigma = np.ones(mesh.nC) * sighalf
xtemp = np.linspace(-150, 150, 21)
ytemp = np.linspace(-150, 150, 21)
xyz_rxP = utils.ndgrid(xtemp - 10.0, ytemp, np.r_[0.0])
xyz_rxN = utils.ndgrid(xtemp + 10.0, ytemp, np.r_[0.0])
xyz_rxM = utils.ndgrid(xtemp, ytemp, np.r_[0.0])


rx = DC.Rx.Dipole(xyz_rxP, xyz_rxN)
src = DC.Src.Dipole([rx], np.r_[-200, 0, -12.5], np.r_[+200, 0, -12.5])
survey = DC.Survey([src])
sim = DC.Simulation3DCellCentered(mesh, survey=survey, sigma=sigma, bc_type="Neumann")

data = sim.dpred()


def DChalf(srclocP, srclocN, rxloc, sigma, I=1.0):
    rp = (srclocP.reshape([1, -1])).repeat(rxloc.shape[0], axis=0)
    rn = (srclocN.reshape([1, -1])).repeat(rxloc.shape[0], axis=0)
    rP = np.sqrt(((rxloc - rp) ** 2).sum(axis=1))
    rN = np.sqrt(((rxloc - rn) ** 2).sum(axis=1))
    return I / (sigma * 2.0 * np.pi) * (1 / rP - 1 / rN)


data_anaP = DChalf(np.r_[-200, 0, 0.0], np.r_[+200, 0, 0.0], xyz_rxP, sighalf)
data_anaN = DChalf(np.r_[-200, 0, 0.0], np.r_[+200, 0, 0.0], xyz_rxN, sighalf)
data_ana = data_anaP - data_anaN
Data_ana = data_ana.reshape((21, 21), order="F")
Data = data.reshape((21, 21), order="F")
X = xyz_rxM[:, 0].reshape((21, 21), order="F")
Y = xyz_rxM[:, 1].reshape((21, 21), order="F")


fig, ax = plt.subplots(1, 2, figsize=(12, 5))
vmin = np.r_[data, data_ana].min()
vmax = np.r_[data, data_ana].max()
dat0 = ax[0].contourf(X, Y, Data_ana, 60, vmin=vmin, vmax=vmax)
dat1 = ax[1].contourf(X, Y, Data, 60, vmin=vmin, vmax=vmax)
plt.colorbar(dat0, orientation="horizontal", ax=ax[0])
plt.colorbar(dat1, orientation="horizontal", ax=ax[1])
ax[0].set_title("Analytic")
ax[1].set_title("Computed")

print(np.linalg.norm(data - data_ana) / np.linalg.norm(data_ana))
plt.show()

Estimated memory usage: 301 MB