SimPEG.maps.ParametricBlock

class SimPEG.maps.ParametricBlock(mesh, epsilon=1e-06, p=10, **kwargs)

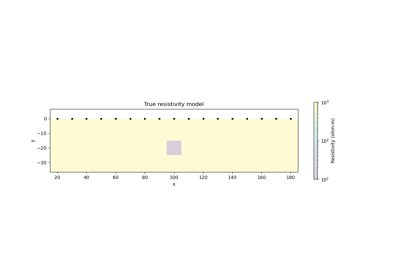
Bases: SimPEG.maps.BaseParametric

Mapping for a rectangular block within a wholespace.

This mapping is used when the cells lying below the Earth’s surface can be parameterized by rectangular block within a homogeneous medium. The model is defined by the physical property value for the background (${\sigma }_0$), the physical property value for the block (${\sigma }_b$), parameters for the center of the block ($x_b[,y_b,z_b]$) and parameters for the dimensions along each Cartesian direction ($dx[,dy,dz]$)

For this mapping, the set of input model parameters are organized:

$$\begin{array}{r}\mathbf{m}=\{\begin{array}{l}1D:[{\sigma }_0,{\sigma }_b,x_b,dx]\\ 2D:[{\sigma }_0,{\sigma }_b,x_b,dx,y_b,dy]\\ 3D:[{\sigma }_0,{\sigma }_b,x_b,dx,y_b,dy,z_b,dz]\end{array}\end{array}$$

The mapping $\mathbf{u}(\mathbf{m})$ from the model to the mesh is given by:

$$\mathbf{u}(\mathbf{m})={\sigma }_0+({\sigma }_b-{\sigma }_0)[\frac{1}{2}+{\pi }^{-1}\arctan (a\mathit{\eta }(x_b,y_b,z_b,dx,dy,dz))]$$

where a is a parameter that impacts the sharpness of the arctan function, and

$$\mathit{\eta }(x_b,y_b,z_b,dx,dy,dz)=1-\sum _{\xi \in (x,y,z)}[(\frac{2({\mathit{\xi }}_{\mathit{c}}-{\xi }_b)}{d\xi }{)}^2+{\epsilon }^2{]}^{p/2}$$

Parameters $p$ and $\epsilon$ define the parameters of the Ekblom function. ${\mathit{\xi }}_{\mathit{c}}$ is a place holder for vectors containing the x, [y and z] cell center locations of the mesh, ${\xi }_b$ is a placeholder for the x[, y and z] location for the center of the block, and $d\xi$ is a placeholder for the x[, y and z] dimensions of the block.

Parameters

meshdiscretize.BaseMesh

A discretize mesh

indActivenumpy.ndarray

Active cells array. Can be a boolean numpy.ndarray of length mesh.nC or a numpy.ndarray of int containing the indices of the active cells.

slopefloat

Directly define the constant a in the mapping function which defines the sharpness of the boundaries.

slopeFactfloat

Scaling factor for the sharpness of the boundaries based on cell size. Using this option, we set a = slopeFact / dh.

epsilonfloat

Epsilon value used in the ekblom representation of the block

pfloat

p-value used in the ekblom representation of the block.

Examples

Attributes

epsilon	epsilon value used in the ekblom representation of the block.
nP	Number of parameters the mapping acts on.
p	p-value used in the ekblom representation of the block.
shape	Dimensions of the mapping

Methods

deriv(m)	Derivative of the mapping with respect to the input parameters.
mDict(m)	Return model parameters as a dictionary.

Galleries and Tutorials using SimPEG.maps.ParametricBlock

Parametric DC inversion with Dipole Dipole array

Parametric DC inversion with Dipole Dipole array

Tensor Meshes

Tensor Meshes

Cylindrical Meshes

Cylindrical Meshes

Tree Meshes

Tree Meshes